Improper Integrals Cheat Sheet
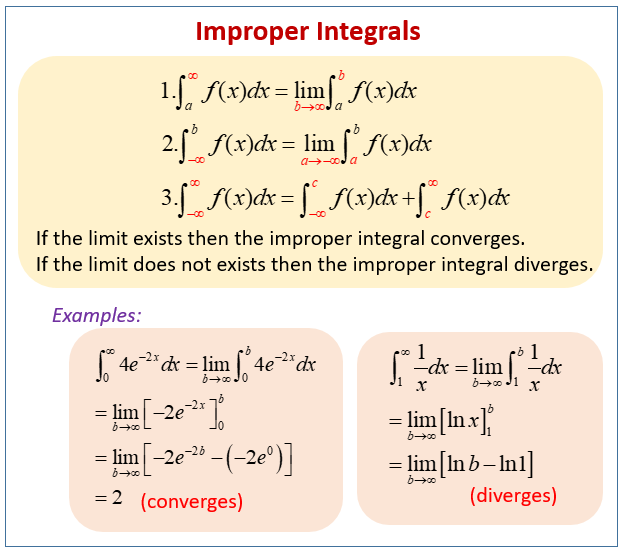
Improper Integrals Cheat Sheet - Improper integral an improper integral is an integral with one or more infinite limits and/or. An integral where one or both. Obtained by rotating the curve y = f (x) over the interval [a, b]. You must separate an integral with an interior infinite discontinuity into two. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot.
Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot. Improper integral an improper integral is an integral with one or more infinite limits and/or. You must separate an integral with an interior infinite discontinuity into two. Obtained by rotating the curve y = f (x) over the interval [a, b]. An integral where one or both.
An integral where one or both. You must separate an integral with an interior infinite discontinuity into two. Improper integral an improper integral is an integral with one or more infinite limits and/or. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot. Obtained by rotating the curve y = f (x) over the interval [a, b].
Modern outline icon of improper integrals 46800147 Vector Art at Vecteezy
You must separate an integral with an interior infinite discontinuity into two. Obtained by rotating the curve y = f (x) over the interval [a, b]. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot. An integral where one or both. Improper integral an improper integral is an integral with one or more infinite limits and/or.
SOLUTION Calculus cheat sheet integrals Studypool
Obtained by rotating the curve y = f (x) over the interval [a, b]. You must separate an integral with an interior infinite discontinuity into two. Improper integral an improper integral is an integral with one or more infinite limits and/or. An integral where one or both. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot.
SOLUTION Improper Integrals Study Guide Cheat Sheet Studypool
Improper integral an improper integral is an integral with one or more infinite limits and/or. Obtained by rotating the curve y = f (x) over the interval [a, b]. An integral where one or both. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot. You must separate an integral with an interior infinite discontinuity into two.
Improper Integrals (examples, solutions, videos)
Improper integral an improper integral is an integral with one or more infinite limits and/or. You must separate an integral with an interior infinite discontinuity into two. Obtained by rotating the curve y = f (x) over the interval [a, b]. An integral where one or both. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot.
SOLUTION Improper Integrals Study Guide Cheat Sheet Studypool
Obtained by rotating the curve y = f (x) over the interval [a, b]. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot. Improper integral an improper integral is an integral with one or more infinite limits and/or. An integral where one or both. You must separate an integral with an interior infinite discontinuity into two.
Calculus Cheat Sheet Integrals Studocu
An integral where one or both. You must separate an integral with an interior infinite discontinuity into two. Improper integral an improper integral is an integral with one or more infinite limits and/or. Obtained by rotating the curve y = f (x) over the interval [a, b]. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot.
L10 Improper Integrals Lecture 11 Improper Integrals MATH 2205
You must separate an integral with an interior infinite discontinuity into two. An integral where one or both. Improper integral an improper integral is an integral with one or more infinite limits and/or. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot. Obtained by rotating the curve y = f (x) over the interval [a, b].
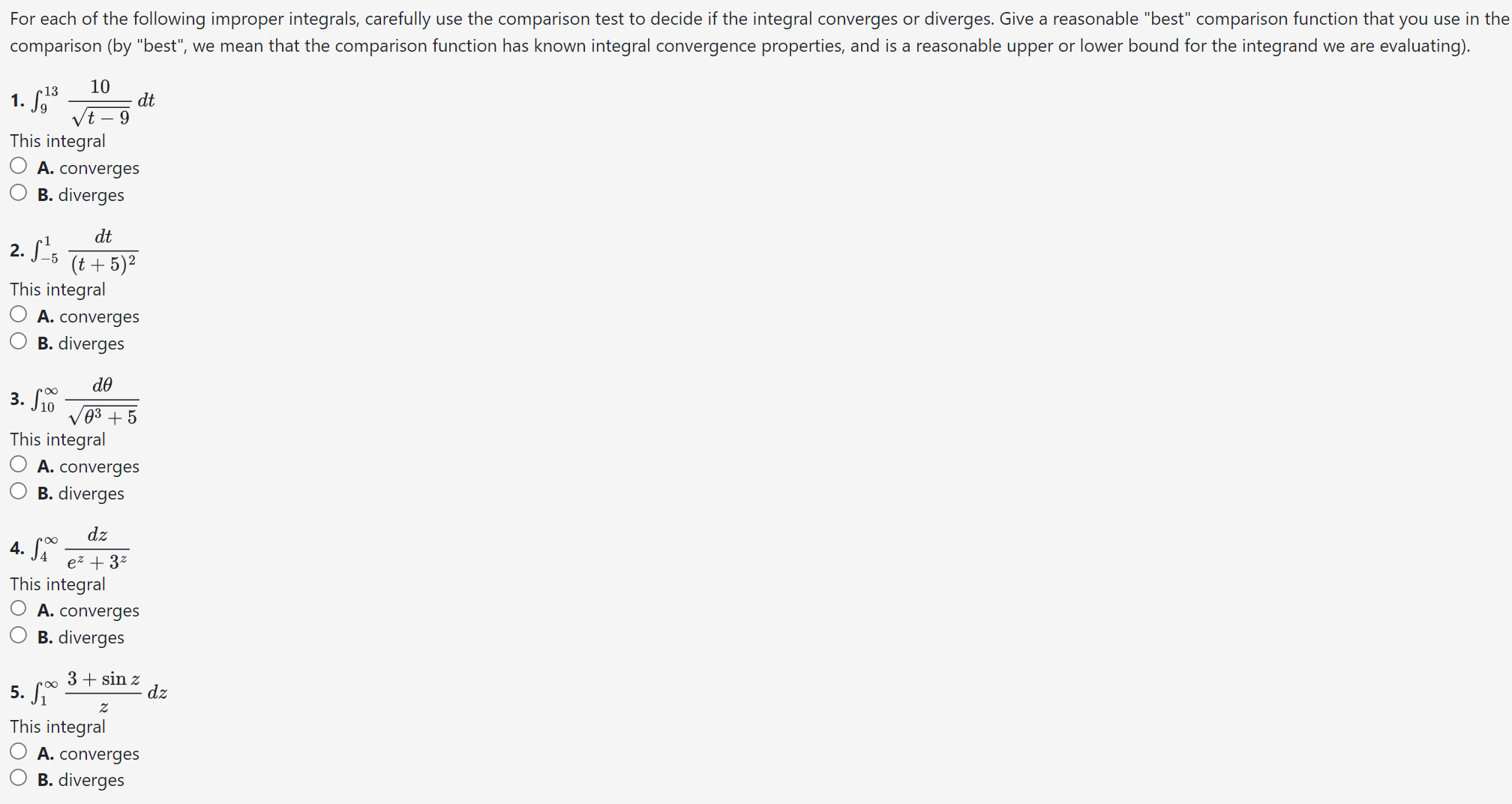
Solved For each of the following improper integrals,
Improper integral an improper integral is an integral with one or more infinite limits and/or. You must separate an integral with an interior infinite discontinuity into two. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot. Obtained by rotating the curve y = f (x) over the interval [a, b]. An integral where one or both.
Improper Integrals
An integral where one or both. You must separate an integral with an interior infinite discontinuity into two. Obtained by rotating the curve y = f (x) over the interval [a, b]. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot. Improper integral an improper integral is an integral with one or more infinite limits and/or.
How To Solve Improper Integrals
Improper integral an improper integral is an integral with one or more infinite limits and/or. An integral where one or both. Obtained by rotating the curve y = f (x) over the interval [a, b]. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot. You must separate an integral with an interior infinite discontinuity into two.
Obtained By Rotating The Curve Y = F (X) Over The Interval [A, B].
Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot. You must separate an integral with an interior infinite discontinuity into two. Improper integral an improper integral is an integral with one or more infinite limits and/or. An integral where one or both.